Casa
four square theorem

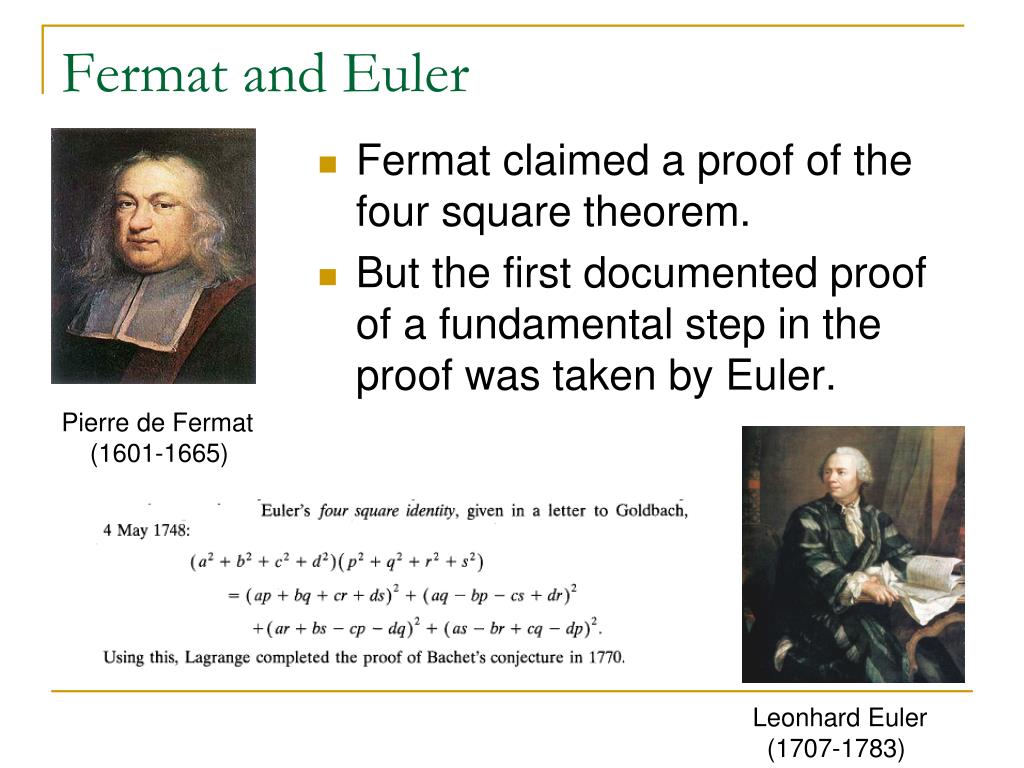
Lagrange's Four-Square Theorem Seen Using Polygons and Lines

Por um escritor misterioso

Descrição
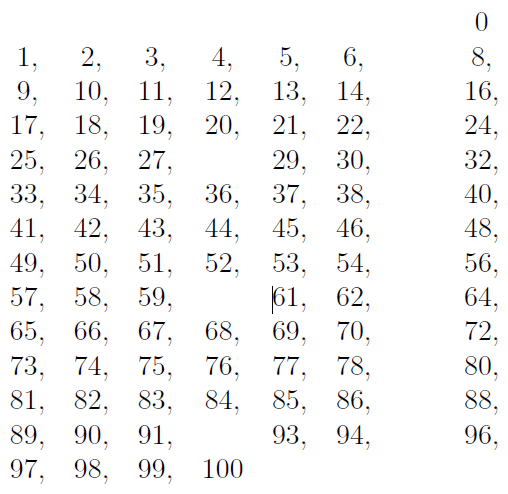
Any natural number can be represented as the sum of the squares of four non-negative integers. For most numbers there are multiple representations. In this Demonstration the four integers (not squared) may be viewed using three different options.1. For each set of four numbers a polygon is displayed with vertices and .2. Each set is sorted from smallest to largest. The first two numbers a;;
Lagrange's Four-Square Theorem Seen Using Polygons and Lines
Lagrange's Four-Square Theorem Seen Using Polygons and Lines - Wolfram Demonstrations Project
Lagrange's Four-Square Theorem Seen Using Polygons and Lines
Lagrange's four-square theorem - Wikipedia
Lagrange's Four-Square Theorem Seen Using Polygons and Lines
Cloud, Newton polygon and some supporting lines of P in (2)
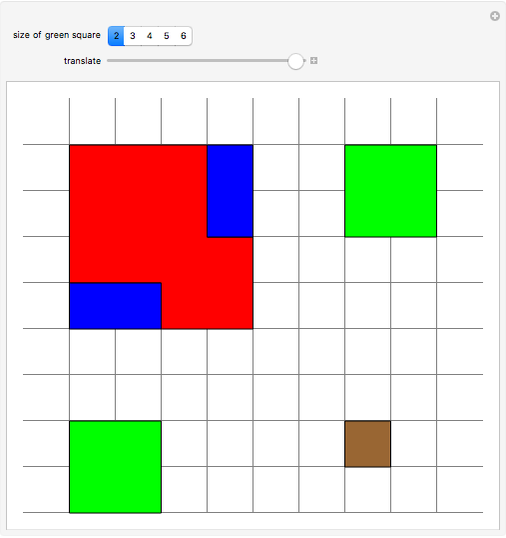
Lagrange's Four-Square Theorem Seen Using Polygons and Lines
Dissecting a Square to Four Squares - Wolfram Demonstrations Project
Lagrange's Four-Square Theorem Seen Using Polygons and Lines
Lagrange's Four-Square Theorem Seen Using Polygons and Lines - Wolfram Demonstrations Project
Lagrange's Four-Square Theorem Seen Using Polygons and Lines
Math Garden: Pascal's Theorem
Lagrange's Four-Square Theorem Seen Using Polygons and Lines
Dotted lines indicate original control polygon while continuous curves
Lagrange's Four-Square Theorem Seen Using Polygons and Lines
MathType - Lagrange's four-square theorem states that every natural number can be represented as the sum of four integer squares. Proved by Joseph Louis #Lagrange in 1770, it can be regarded as
Lagrange's Four-Square Theorem Seen Using Polygons and Lines
Examples of discontinuous quadratures over irregular convex polygons
de por adulto (o preço varia de acordo com o tamanho do grupo)